Experiment : alternative versions of the Chartres Labyrinth

Chartres Labyrinth - How many different versions can we create ?

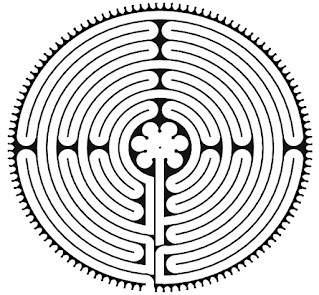
I recently stumbled upon a famous labyrinth in Chartres (France), 12th century.

At first sight, Chartres Labyrinth is amazing. You can't immediately figure out how it works (entrance, exit, path, dead ends, ...). It appears on the paved floor of a religious building. When I was there, the labyrinth was visible under numerous chairs installed on the floor. In those circonstances, you try to follow the path of the labyrinth with your eyes, from a distance. But its concentric circles have a puzzling visual effect. You need to follow it for real, on foot.

After a while, trying to follow the path, avoiding the chairs, it appears that it is not a maze : it has only one path, no dead ends, the entrance and the exit are the same. The specialists use the term "unicursal".

Then a question arise : how many of those labyrinths can you create, following the same design and rules (more or less). If there are more than one solution, why choose this particular one.

This question can take a bit of time.. I guess mathematicians know the answer.
I finally managed (hardship with design softwares...) to produce some variants of the Chartres Labyrinth. There seem to be a lot more...

That could be the subject of a playful hoax : flooding the internet with images of alternative versions of the Chartres Labyrinth, and see if people get caught. Am I just trying that here, on a modest scale ?

Some alternative versions to the Chartres Labyrinth - 4 sectors - 11 circuits

this one has a small dead end and a short straight path that is an island ; 

not perfect, because all the surface is not used

This one has 2 small dead ends : we should avoid that

this one contains a closed curved path that is an "island" (in the middle of the down right quarter)  : 

an example of what we should certainly avoid

this one is better, but still has a small dead end, and a straight island

this one is cool... only 2 small dead ends 

but several branches to and from the central trunk

Same version, but without the dead end

Some alternative versions, Chartres-like Labyrinths - 5 sectors - 11 circuits

Not that bad, with five lobes, one dead end here

Two small dead ends, here

Same as before, but without the dead ends, 

feels a bit unbalanced ?

Chartres like Labyrinth carved on a Tagua nut - finger labyrinth - hypnoglyph

The same labyrinth as before, carved on a Tagua nut, 

to be used as a finger labyrinth

Chartres like Labyrinth drawn on a spherical surface - Potato

Another 5 lobes labyrinth, wrapped onto a potato :

The potato was granted the following version 

of a five lobes Chartres-like Labyrinth :

Transposing - Chartres-like Labyrinth - Dodecahedron - 5 sectors - 11 circuits

The same version as before, after a transposition (by different method than for the potato) on the surface of a dodecahedron.

There, each circuit/level is represented by one face of the dodecahedron, among eleven of those. The last face represents the inner and the outer space (center and outside of the Chartres-like labyrinth). Each lobe, and each of the two central paths of the Chartres-like Labyrinth (the entry and arrival at the centre)  is represented by a lane in which the path will be followed.  

Here a video silently illustrating (or just suggesting) the process of transposition :

Another Chartres like Labyrinth carved on a Tagua nut - finger labyrinth - hypnoglyph

The next 5 lobes, 11 circuits Chartres-like labyrinth I'll make will be this one :

here, carved on a rather angular tagua nut

Computer program generated Chartres-like Labyrinths - javascript - Google Script

 June 10, 2020 - I finally wrote my own prototype of a labyrinth generator computer program... it's still imperfect, limited by my incompetence and by some restrictions of the tools i could use (exploration with Google script + rendering in html and javascript). With Google script, despite the 6 minutes limit of running time, the whole exploration is possible for 11 circuits and 5 sectors (with some pruning to avoid disgraceful labyrinths)... but not all of the output via the log (don't know how to write elsewhere, yet). For the rendering, I've some improvements to do...

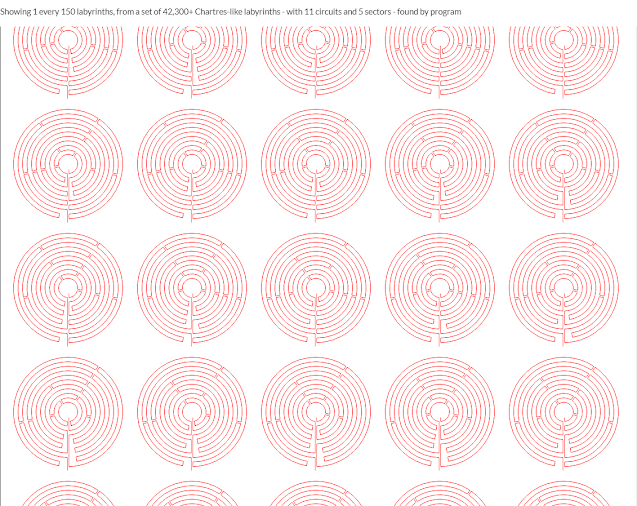
Some of the 42300 labyrinths found by the computer program

See here, 1 in 150 of the more than 42300 labyrinth the computer program has found. :

 https://sites.google.com/view/labyrinths-examples/labyrinths-11-circuits-5-sectors-1-in-150

42341 labyrinths and a strange technical glitch

See how the technique used (canevas, in html) draws labyrinths that are more "decayed" when drawn farther from the origin.

click on the video below...

More info found : what other people had been doing...

Blogmymaze - Andreas Frei

April 10, 2020 - I find a blog, BLOGMYMAZE ( https://blogmymaze.wordpress.com ), that explores labyrinths, and examines a few cases of "11 circuits, 4 sectors" labyrinths :

https://blogmymaze.wordpress.com/2018/06/03/the-complementaries-of-the-three-very-interesting-historical-labyrinths-with-4-arms-and-11-circuits/

Mark Wallinger

April 10, 2020 - Someone has already done this kind of work extensively : in 2013, Mark Wallinger, British artist, has shown 270 distinct labyrinths, one in each of London tube station. There was also a catalog of that; see for instance : 

https://bloggermymaze.files.wordpress.com/2015/10/tube_book_02.jpg?w=400&h=300&zoom=2

Jo Edkins

May 8, 2020 - An interesting blog compares existing (or having existed) mazes similar to the Chartres Labyrinth. It uses the rectangular transcription to ease the comparison.  

Also shows some new designs. http://www.gwydir.demon.co.uk/jo/maze/chartres/index.htm ... this site will soon cease to exist, replaced by www.theedkins.co.uk .... where I was unable to find the same information about mazes... too bad...

Caerdroia - Labyrinthos - Andreas Frei, Hellen Galo, Tristan Smith, Jacques Hébert, ...

May 24, 2020 - Publications n°33 to n°39 of "Caerdroia - the Journal of Mazes & Labyrinths" are available in PDF versions on http://www.labyrinthos.net/digitaldownload.html. Particularly, among many other things, you'll see articles about :

- some variants of Chartres-like labyrinths, explanation from Andreas Frei about his way of cataloging labyrinths, in Caerdroia 39  (links to his website, in German, that seems rather empty) ; 

- Amerindian mazes, in Caerdroia 38 ;

- how to create perfect labyrinths (Frei's graph + Hébert notation + 6 canonical labyrinths),by Ellen Galo, in Caerdroia 37 ; this article is also available in the archive of the most requested past articles... so... the subject is clearly interesting to a fair number of people :-)

- Kota labyrinths in southern India, in Caerdroia 36 ;

- how to analyze, design and scale up chartres-like labyrinths, by Andreas Frei, in Caerdroia 35 ;

- a computer program for generating medieval labyrinths, by Tristan Smith, in Caerdoia 35; we read that without enforcing strict enough rules about the properties of the labyrinths, there is ~5 million versions of a 11 circuits / 4 sectors labyrinth. The article links to Tristan Smith's website, where a good number (rapid count : more than 130) of alternative versions of Chartres-like labyrinths have been generated by the program (or with its help), using strict enough rules. The set of rules of Jacques Hébert gives just 20 "canonical labyrinths" that would be (i guess) the most "Chartres-like".

See  https://www.otsys.com/~tsmith/daedalus.html ; the images of the labyrinths are in 4 pdfs ;

Also, the definitions corresponding to the rules that the program can respect are here  https://www.otsys.com/~tsmith/properties.html (but the article in Caerdrioa is clearer : shows examples)

- a mathematical notation for medieval labyrinths, by Jacques Hébert, in Caerdroia 34;

surprise... Andreas Frei is actually also one of the authors of the blog Blogmymaze (see higher)... 

The most requested past articles of Caerdoia are made available in the archive

  http://www.labyrinthos.net/caerdroiaarchive.html#CaerdroiaIndex which also has a PDF listing all of the articles from 1980 to 2019... from which i extract this very small selection : the articles that may help in describing and creating variants :

#29 (1998)	Developing the Labyrinth: Alex Champion, p.43-51: re-drawing the classical labyrinth - new variants.
#34 (2004, pdf online)	A Mathematical Notation for Medieval Labyrinths, p.37-43: Jacques Hébert explains.
#35 (2005, pdf online)	The Cascading Serpentine, p.19-26: Andreas Frei examines the Chartres labyrinth structure.
#35 (2005, pdf online)	A Daedalus for the 21st Century, p.27-33: Tristan Smith’s software labyrinth builder.
#37 (2008, pff online)	Sigmund Gossembrot’s Labyrinth: A Very Special Design, p.41-44: Andreas Frei takes a look at an unusual labyrinth from the 15th century.
#37 (2008, pdf online)	Further Thoughts on ‘Perfect’ Labyrinths & How to Create Them, p.45-49: Ellen Galo dissects the structure of mathematically ‘perfect’ labyrinths.
#38 (2008, pdf online)	Two Labyrinths Compared: What They Have in Common, p.60-63: Andreas Frei takes a look at two apparently different labyrinths in early manuscripts.
#39 (2009, pdf online)	The True Design of Sens, p.28-32: Richard Myers Shelton compares the two known designs of the Sens Cathedral labyrinth and asks which is correct?
#39 (2009, pdf online)	A Catalogue of Historical Labyrinth Patterns, p.37-47: Andreas Frei describes the findings of his labyrinth design analysis project. Labyrinthos Archive 13
#40 (April 2011)	Greys Court: an invitation to symmetry, p.21-35: Richard Myers Shelton explores the symmetry inherent in certain labyrinths.
#40 (April 2011)	Considering the Duality of Labyrinths, p.40-47: Andreas Frei examines a hidden property of labyrinth designs.
#46 (July 2017)	Searching in the Mirror, p.34-48: Richard Myers Shelton completes his series on the geometry of symmetric labyrinth designs.
#48 (April 2019)	Basic Labyrinth Math, p.37-49: Richard Myers Shelton explains the rules of labyrinth structure.
#49 (May 2020)	Medieval Marvels: Fifty-Three Eleven-Circuit Manuscript Labyrinths, p.8-27: Jill K.H. Geoffrion & Alain Pierre Louët look at an extensive group of manuscripts produced prior to 1500

2020/09/13 - another description - with stricter rules - of what should be considered a Chartres-like labyrinth. In http://www.lavigne.dk/labyrinth/e6charst.htm. Chartres-like labyrinths would be made of a combination of those patterns :

to be continued....

Experiments : trying to locate the position of the sun - Sunstone - Iceland Spar - Optical Calcite -

How to find the sun with a viking sunstone

We find various claims asserting the sun can be located, when not visible because of the clouds, with an Iceland Spar crystal. Some show experimental videos and conclude that their experiment proves that. I couldn't replicate the experiments. More precisely, I could replicate the experiment, but i couldn't see how it proved that the sun was actually located. 

Conclusions of my first experiments 

• so far, no obvious way to tell which side is the sun ...
• the sun is on the left or on the right, when "maximum" light polarization is detected
• brainstorming for further tries [apparently, almost all nonsense : see "More information", lower]: 
• let light enter from different faces of the crystal;
• really go do the experiment where the Vikings were said to use those crystals for navigation : North Sea, high latitude, on a boat ;
• use refraction ; shape differently the hole that lets the light in ; use 2 crystals ; 
• use reflection on the inside of the faces of the crystal;
• use crystals with various kind of  inhomogeneities, giving complementary information ;
• for example, explore the changes in colors and patterns in some crystals ;
• detect the polarization from under the surface of the water;
• ignore light polarization and the "Raleigh sky model", find some other model... (because the Raleigh sky model seems to have a symmetry that wouldn't allow to decide which side is the sun); 

Videos of the my experiments :

More information

Not Calcite, but Cordierite, tourmaline, ... ?

Read on https://royalsocietypublishing.org/doi/10.1098/rsos.150406

"the Viking sunstones described in the old sagas could have been dichroic cordierite, andalusite and tourmaline or birefringent calcite (Iceland spar) crystals that could serve as linear polarization analysers."

Read on https://royalsocietypublishing.org/doi/10.1098/rsos.150406

"real calcite crystals also have disadvantages, and thus cordierite and tourmaline can also be at least as good sunstones."

Prerequisite step : calibrating and marking the crystal ?

Read on https://royalsocietypublishing.org/doi/10.1098/rsos.150406

[meaning not immediately clear for the lay person !]

"— Calibration step: In cloudless weather [...] rotate (adjust) the crystal until its well-determined orientation (e.g. minimal or maximal intensity of skylight transmitted through a dichroic sunstone, or minimal or maximal intensity difference between the two slots/spots of a birefringent sunstone), where it was fixed, and thereafter he calibrated the crystal by engraving the direction pointing towards the sun on the crystal surface. 

— Navigation step 1: Applying this sunstone rotational adjustment under a cloudy or foggy sky at two different celestial points, the navigator could determine the directions perpendicular to the local E-vectors of skylight shown by the engraved straight markings of the sunstones, pointing towards the sun.  [?? how many sunstones are needed ??]

— Navigation step 2: The intersection of the two celestial great circles crossing the sunstones parallel to their engravings gives the position of the invisible sun." [?? celestial circles crossing the sunstones ??]

Process for calibrating an Iceland spar (calcite)

"the calcite is rotated until the intensity difference between the two light spots is maximal. This occurs four times with 90° periodicity during a full 360° rotation of the crystal. [...] the Viking navigator [...] has to scratch only one straight mark (pointing towards the sun) onto the sunstone, and this sun mark can be used under all weather conditions to determine the position of the invisible sun."

You need two sun stones, not only one !?

Read on https://www.osapublishing.org/josaa/fulltext.cfm?uri=josaa-31-7-1645&id=294365

[another description, where we see that 2 sunstones are involved. 
And we still have to find a proposal about how you perform that on a narrow crowded ship... maybe here, if you want to pay for access : https://www.osapublishing.org/ao/abstract.cfm?uri=ao-52-25-6185 ]

"Step 1 (Fig. 1A): Viking navigators are assumed to have determined the direction of skylight polarization in at least two celestial points with the use of two sunstones to estimate the position of the sun occluded by cloud/fog or being below the horizon. [...] 

Fig. 1A

Step 2 (Fig. 1B): A short scratch on each sunstone could help the navigator to set two celestial great circles across the two investigated sky points parallel to the scratches being perpendicular to the local direction of skylight polarization. Then the navigator determined the above-horizon intersection of these celestial circles. According to the Rayleigh theory of sky polarization [9], this intersection coincides with the position of the invisible sun.

Fig. 1B

Step 3 (Fig. 1C): [... hypothesis for finding the geographical north ...]  "

[better explained here, maybe, for who accepts to pay for science  : https://www.osapublishing.org/ao/abstract.cfm?uri=ao-52-25-6185 ]

Other hints to evaluate 

using 2 sunstones

in  https://www.sciencedirect.com/science/article/abs/pii/S0262407915605553

Haidinger's brush : the easy / feasible method to test ?

Read on https://www.seeker.com/legendary-viking-sunstone-navigation-solved-1765489280.html

"if you look through the crystal in its depolarizing position and then pull it away suddenly from your line of sight, you can catch a glimpse of a faint, elongate yellowish pattern known as a Haidinger's Brush. The key here is that the ends of that yellow shape point directly toward the sun."

[ The thing is not self-explanatory... there are two opposite "ends" in this elongate pattern... and they can't both point directly toward the sun... ]

The study from where this assertion comes from is here:
https://royalsocietypublishing.org/doi/10.1098/rspa.2011.0369
[i'll need to try harder to understand the paper, because at first look, i can't see why we should find the direction of the sun with the Haidinger's brush... which is not directional... we find in the study this beautiful illustration, that is not as convincing as it seems to be

if you manage to see that magnificent Haidinger's brush, you can still rotate 180° and do the experiment again, then... would you not assert that the sun is in the opposite direction ? ]

Navigation by lead and line, and other means

Was it even necessary to locate the sun, the north or any direction ?

Read here how navigation by lead and line was used to determine where the boat was
https://www.cambridge.org/core/journals/journal-of-navigation/article/early-navigation-in-the-north-sea-the-use-of-the-lead-and-line-and-other-navigation-methods/EDA8012AE267C583E8F2EA14EE36E145/core-reader
[ok, not doable when crossing or exploring unknown deep seas]